Morning before the test

Well isn't this strange that I nearly forgot to blog. Hahaha. Why does this keep on happening to me. Well I like to say that this unit was fun in the way that it was. Hahaha. Craig. Hahaha. What a guy. Finishes the logic puzzle and then the second logic puzzle next peroid. Hahaha. Wow. You have to do this to us you know. Hahahaha. Well anyways.... Um.. Back to studying I guess. Hahaha... and Good Luck you guys.

blog before the test

I think this unit is the shortest unit and the one that i enjoyed the most. I think its fun solving the Logic Problems. But unlike Crazy Craig,who can finish it in less than 25 mins., it takes me awhile to figure them out. The one that i liked the most is the one with the forensic experts. The uh, Kansas one. ^_^ pretty cool. I like this Unit because its the easiest unit so far. haha. At first, i didnt really understand the converse, inverse and contrapositive because i was away when they talked about it in class but Mr. K's post helped me alot. ahah. Doing the Venn Diagrams are fun too. The thing that im having a little difficulty with is the indirect reasoning but i still think that this unit is fun.


GOODLUCK ON THE TEST YOU GUYS. ^_^

Getting Ready For The Test

A few links you may find useful in preparing for your test on Thursday:

• Venn Diagrams


• Conditionals (If ... then ...)


• Biconditionals (... if and only if ...)


• Related Conditionals (the other ones)

And here are a few quizzes (refresh the page for more) ...

• Inductive Reasoning and Conjecture (5 questions)


• Conditional Statements (5 questions)


• Venn Diagrams Self Test (13 questions)

And finally, here are some logic puzzles to practice with ...

• Dude Ranch Blues


• Afterschool Activities


• Monkey Business


• Farmyard Pandemonium


• What did you order?

Study hard and do your best work; work you're proud of!

Logical Acrostics

Here's the new set of acrostics for you.

Blogging Prompt
Your task is to create an acrostic "poem" that demonstrates an understanding of logic related to any one of these concepts:

REASONING
CONDITIONAL
COUNTEREXAMPLE
VENN DIAGRAM
DEDUCTION
INDUCTION

As an extra challenge (worth an additional bonus mark) try to make a Double Acrostic, that is, each line should begin and end with a letter of the word you are working with.

Remember, this is a bit of a race. Your answers have to be posted to the blog in the comments to this post. If someone has already used a word or phrase in their acrostic you cannot use the same word or phrase. i.e. It gets harder to do the longer you wait. ;-)

Here is an example of an acrostic that Mrs. Armstrong wrote:

Always in 2 dimensions
Region between the boundaries
Entire surface is calculated
Answer is in units²

Be creative and have fun with this!!

Sunday 3x the Funday

A triple header this weekend.

Mr. Zhong Kui will make you laugh. I think his "problems" are the easiest ones to solve.

Rat is another "escape" puzzle. Every time you do something wrong he squeaks.

No. 5 is a set of three puzzle/adventures to get a little boy out of trouble.

Have Fun!

SCRIBE.

late post? yes, i know... IM REALLY SORRY =(

hmm what happened in class yesterday?
okay,when we got to class Mr. K asked us to draw a BIG circle on a piece of paper. Before we started our activity he mentioned that "Math is science of patterns".

Then Mr. K started off by asking us... "If we connect two points (along the circumference of the circle) how many regions will the circle be divided into ?"

We all know that if we connect two points then it will be divided into 2 regions.

"how about 3 points connected all together?"
he drew on the board three points along the circumference of a circle then counted the number of regions. 4 regions.

and then he asked us to choose 4 points along the circumference of the circle, connect it and count how many regions the circle was divided into. 8 regions.

then we made a chart that looked like this:

looking at the table/chart, is there a pattern? yes there is..

what is the pattern? the number of regions goes up by multiplying 2 to it.

then Mr. K asked us to guess what the next number would be.

"16" the students answered...

is that correct?

well, we have to check if it is...so we drew 5 points along the circumference of the circle and counted the number of regions.

16! we were right !

Then Mr. K asked if the number of points was "n", can we come up with a formula for it?

one of the students said " if n represents the number of points then the number of regions will be 2^n-1.

why "n-1"?

n-1 because the exponent of the number of regions is always one less than the number of points.

Then Mr. K asked what if we have 6 points connected all together? how many regions will the circle be divided into?

using the formula : 2^6-1 or 2⁵ we got 32.

and just like before we have to check... so we drew a circle with 6 points connected to each other then counted the regions.

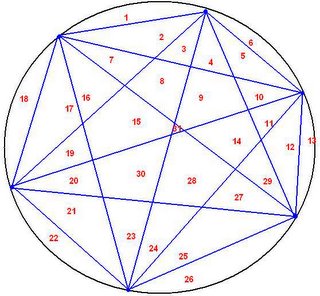
31 regions ?! only 31. That means that the formula we came up with is not true and must be discarded.(some may have gotten only 30 regions. why? maybe you just missed counting a little region or you drew your circle too many) notice that there`s a little triangle region in the middle of the above diagram? if you drew your circle big enough then it should show.

Therefore 6 is the counter example for the formula 2^n-1.

coun·ter·ex·am·ple;

-an example that disproves a hypothesis, proposition and/or theorem.

"PRIME PRODUCING" POLYNOMIAL

F(x)=x²+x+41

is this really a prime producing formula? let`s check it out.

F(1) = 43 prime

F(2) = 47 prime

F(3) = 53 prime

F(4) = 61 prime

F(5) = 71 prime

okay, so far all the result are prime numbers but is it right to conclude that f(x) will be prime for ALL intergers?

answer: NO

why?: because if you try to solve it with x = 40

F(4o)=4o²+4o+41

F(40)=40(40+1)+41

F(40)=1681 which is also 41²

MARIN MERSENNE.

(picture taken from:http://shl.stanford.edu/Eyes/kircher/mersenne.html)

Marin Mersenne is a french theologian, natural philosopher, and mathematician who tried to find a formula that would represent all primes but didn't succeed. Although he failed, his work on numbers of the form
2^{p - 1}

p prime

is still of interest in the investigation of large primes.

He's name is best remembered today for Mersenne Prime.

Mersanne Prime: are primes of the form 2^p-1.

For more about Mersanne Primes: *click_here* or here

(it includes a table of known Mersenne Primes, who discovered it and when)

So far they have only found 42? mersenne primes.

Wanna become world famous? or even win some cash? You can participate in what they call GIMPS. Great Internet Mersenne Prime Search. They have already found seven of them on GIMPS.

If you're interested you can CLICK_HERE. It tells you how it works, how long it will take, how much you can win, what you need to have, EVERYTHING you need to know. :)

for more about Marin Mersanne clickhere

VENN DIAGRAMS

Mr. K also posted up some questions on the board.

1.) In a group of students 12 are taking chemistry, 10 are taking physics, 3 are taking both and 5 are taking neither. How many students are in the group?

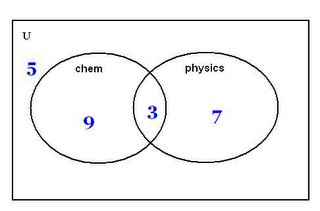
ANSWER: 24 students are in the group

2.)In a third-rate rock band, 3 people play guitar, 4 sing, 2 do both and 6 have no talent for singing or guitar so they do something else. How many are in the band?

ANSWER:There are 11 people in the band.

3.)There are 64 kids in the "Tiny Little Cherubs"(TLC) Daycare. At lunch 59 ate green beans, 56 cauliflower, 60 ate brocolli, 55 ate green beans and cauliflower, 54 cauliflower and brocolli, 56 green beans and brocolli and 53 ate all. How many ate none?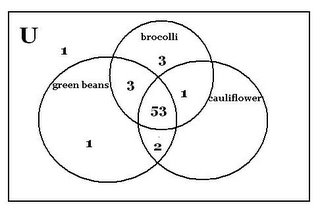

ANSWER: One kid ate none.

OKAY... maybe i exaggerated when i said it wasnt half way yet -_-". lol. hhm, thats all i could remember...so i guess this is it.

THE EEND :)

sorry again for being late!
*if i missed anything please let me know*

oh and i found a cool site about prime puzzles and problem connected. I don't have a delicious account so i guess ill just link it in here.

primepuzzles

There's a new puzzle every saturday and the solutions will be up one week later.

oh... the scribe today was jamilynG. check out her scribe post for tomorrow's scribe.

Sunday Jumping Funday!

'The goal of the puzzle is to switch the the pegs on the left with the pegs on the right by moving one peg at a time.

Move pegs by clicking and dragging them to open slots. A peg may only be moved to an open slot directly in front of it or by jumping over a peg to an open slot on the other side of it. You may not move backwards. The game ends when you win or get stuck.'

Play the game here. Can you win the 8 peg game? ;-)

(Thanks again to Think Again!)

Blog. Test tomarrow... Blog

Well I guess it's me who nearly forgot to blog. Hahaha. So lazy these days... So anyways. Well Let's see. This unit is... Confusing. Well sorta. I get it and all that but you know it's all good. Yeah. Well I know most of the concepts so I'll be fine. Becides this week has been so crazy. With the math Assigment and all that. Wooo Nearly forgot. Eh Robert. Hahaha. Well I hope that I do good on the test. Good luck to all of you too. Hahahaha. Well I got nothing else to do. Maybe some studying would do me good... Anybody up for an all nighter on the chat box. lol.

My Acrostic (tangent)

Tangent is a straight line that touches a circle but does not cut it.
Any tangent line to a circle is perpendicular to the radius drawn to the point of tangency.
Not only a line but it is also a trig function.
GA (line) is a tangent. Therefore, the angle between tangent GA and chord BC (angle1) is congruent to the inscribed angle subtended by the opposite side of the chord(angle 2).
EG (line) and GB(line) are congruent because they are drawn from the same point.
Note that in the case of a circle, the tangent line will touch it ONLY at a single point.
Tangent and radius create a 90 degree angle when they intersect.


.

All Together Now!

OK folks, just like we did in class today. Everybody help each other and and solve this one in the comments to this post -- it's good practice for your test. ;-)

scribin' at the last minute...

* sorry guys i did this at the last possible time i was suppose to work until 10:00pm ended up working until 11:00pm.

- Well, today we did a quiz on our circle geometry. Here are the questions on the quiz so you can try it again or for the first time if you were not there. In the end I'll post up the answers.
.............................................................
Circle Geometry Quiz 2

(1) angle BOC = 115'. Find the measures of arcs AB, BC, CD, and AD. Justify your solution (4 marks)

(2) If the measure of chord AB is 14cm and the measure and the measure of chord DC is 7 cm, how far from the centre of the circle is chord DC? Justify your solution. (4 marks)


(3) How far is a chord of length 8 cm from the centre of a circle with a diameter of 10cm? (2 marks)

(4) What is the diameter of a circle in which a chord 16 cm long is 15 cm from the centre? (3 marks)

..........................................................................................

ANSWERS:* i wish i could've made it into columns

(2) STATEMENT / REASON
AB = 14 cm / GIVEN
DC = 7 cm / GIVEN
OB = 7 cm & AO = 7 cm / RADII, AND AB DIAMETER
DE = 3.5 cm & EC = 3.5 cm / PERPENDICULAR BISECTOR THEOREM
OC = 7 cm / Radius
OE = 6.06 cm / PYTHAGOREAN THEOREM

a^2 + b^2 = c^2
3.5^2 + b^2 = 7^2
12.25 + b^2 = 49
49 - 12.25 = b^2
b^2 = 36.75
b = 6.06

(3) PYTHAGOREAN THEOREM
a^2 + b^2 = c^2
4^2 + b^2 = 5^2
16 + b^2 = 25
25 - 16 = b^2
b^2 = 9
b = 3

The chord is 3 cm away from the centre of the circle.

(4) PYTHAGOREAN THEOREM
a^2 + b^2 = c^2
15^2 + 8^2 = c^2
c^2 = 289
c = 17
diameter = 17 x 2 = 34 cm

The diameter of the circle is 34 cm.

............................................................

* PHEW.... sorry again guys well next scribe will be....

rannell d.

hah =) have fun rannell.

Cubeoban Sunday

The objective of Cubeoban is to push/pull all the blocks to their corresponding lights. Do this by clicking on the blocks and drag them in the direction you want to push them. Play it here.

Level 1 was so easy that even I could do it. Level 2 (the image), started my thinking.

(Thanks again to Think Again!)

Circle Geometry Review

We're gearing for our test now. Here's a bunch of online quizzes you can use to help get ready. Also, check out the links in our del.icio.us box, there are some really good ones there. ;-)

• Proof of Congruence (5 questions - refresh the page for more quizzes)


• More Congruence (5 questions - refresh the page for more quizzes)


• Circles (5 questions - refresh the page for more quizzes)


• Arcs &Angles (5 questions - refresh the page for more quizzes)


• Arcs & Chords (5 questions - refresh the page for more quizzes)


• Inscribed Angles (5 questions - refresh the page for more quizzes)


• Tangents (5 questions - refresh the page for more quizzes)


• Polygons (5 questions - refresh the page for more quizzes)

Whew! More quizzes than you can shake a stick at! ;-)

Scribe #48 I think... Nah... Make it 50.

Well... Long time no Scribe... Hahaha. lol Anyways... Today's Chapter is...

One Regular Day in MATH

Today was a regular day. One day too many. Yet one day too late.

"Hi guys" I said as I walked in the room. I was so happy yet so sad... It's math once again and I feel so bad. It's math. It's math. I am so very pleased. Yet I still rhyme. Get to work... Geez...

Ok. Ok. I looked happily. Three problems on the board. Three problems for we.

(Ok I'll stop I have a Poetry English Assignment... Ok. Hahaha…)

1. Find the values of x and y. Justify your answer.
Hint.… The checkmarks and the o's are congruent.

So we all do the really long way.… Then Mr. K. Tells us to check our dictionary's

ALLWAYS BRING YOU DICTIONARY TO CLASS!!!!

All of your tools are there. lol

So to justify you have to use the Statement/Reason that we learned. He showed us that we could use the Exterior Angle Theorem.

Statement Angle DEF = 70º X = 100º Y = 60º

Reason Ext. to Triangle ADE Angle CEF is EXT. to Triangle ACE Angle ACE is EXT. To Triangle ABC

2. For each pair of triangles write the propeterty which proves the triangles are congruent.



For this one... You had to look in your dictionary for one of four propeteries: SSS, SAS, AAS, or RHL. Which translates to: Side-Side-Side, Side-Angle-Side, Angle-Angle-Side and Right Angle-Hypotonuse-Leg. By the way you are only allowed to use those four propeteries.

Also Mr. K. Taught us the Reflexive Property. Quote "In the reflexive Propetry. You are the same thing as yourself." Basicly If you have a diagram and cut it directly in the middle. One half of the diagram is the same as the other half.

Well in the end you should of got (from left to right, top to bottom) RHL, SSS, NOT CONGRUENT, AAS, SAS, AAS

3. Find two congruent triangles and explain why they are congruent.

This one was a mix of the first question and the second question. So it was really hard. Hahaha...

In the end it ended up like this:

Statement Line Segment DC is Congruent to Line Segemnt BC Angle BCD is Congruent to Angle DCB Line Segment AC is Congruent to Line Segment EC Triangle ACB is Congruent to Triangle DCB

Reason Given Reflexive Propetry Given SAS

There was another way... To it to. It involved the top triangles and the congruent angles in the middle

After that he gave us two more questions. What fun. Ha ha ha...

To be honest I really forgot how to do these questions... The bell rang when we were going to review them and I was so lost when I was doing them... Ha Ha Ha?

Anyways... Homework is Excerise 35

AND THE NEXT SCRIBE IS...
It reads Kasia... Hahaha.

By the Way... All Math images are made by EUKLID.
Provided By Graeme. ;)

ALSO... I really think there's a PRE-TEST tomarrow... Look's Up...